miliblast.blogg.se - Things to know about sas geometry

RHS rule states that if in a right angled triangle hypotenuse and one side are equal, the two triangles are congruent. The angle between any two sides of a triangle is called the included angle of the triangle.use The Law of Cosines to calculate the unknown side, then use The Law of Sines to find the smaller of the other two angles, and then use the three angles add to 180 to find the last angle.

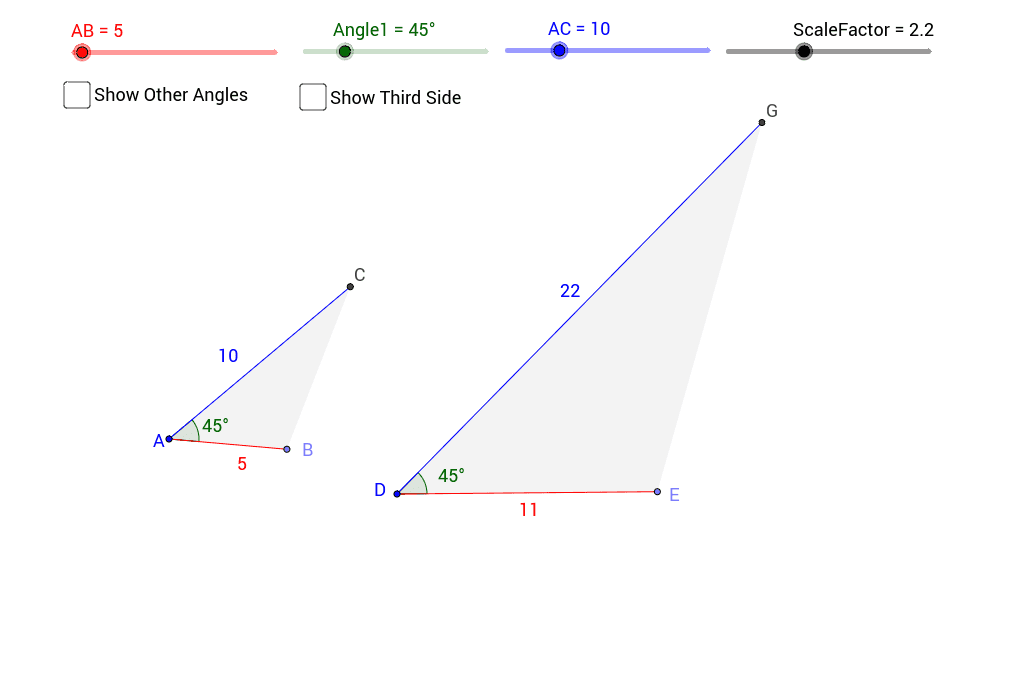
ASA congruence rule states that if two angles and a side in the middle of the two angle are equal, the triangles are congruent ' SAS ' is when we know two sides and the angle between them.SAS congruence rule states that if two sides and an angle in the middle of the two sides are equal, the two triangles are congruent.SSS congruence rule states that if all sides of a triangle are equal, the triangles are congruent.There are 4 rules to determine if two triangles are congruent: SSS, SAS, ASA, RHS.Congruent triangles are those triangles whose sides and angles are exactly equal.If all the three corresponding sides of two triangles are equal then they are said to be congruent by SSS rule. RHS (Right angle- Hypotenuse-Side)- If the hypotenuse and side of one right-angled triangle are equal to the hypotenuse and the corresponding side of another right-angled triangle, the two triangles are congruent.AAS(Angle-Angle-Side)- Two triangles are said to be congruent by AAS condition if their two angles and 1 side are equal.ASA(Angle-Side-Angle)- Two triangles are said to be congruent by ASA condition if their two angles and 1 side are equal.SAS(Side-Angle-Side)- Two triangles are said to be congruent by SAS condition if their two sides and 1 angle are equal.SSS (Side-Side-Side) – Two triangles are said to be congruent by SSS condition if all three sides are equal.

If there are two triangles A and B then if they fulfil any of the below mentioned conditions then they are said to be congruent and they are mentioned like below:

For example: Because you were able to prove that two sides with their included angle were congruent, you would use side-angle-side to prove that the triangles are congruent.So, we can say both triangles ABC and PQR are congruent. If you have a second triangle that also has side lengths 5 and 10 with a 30 degree angle in between, then by the SAS Postulate we know that the two.

Hypotenuse leg (HL): the hypotenuse and one leg of each triangle are equal.

Angle-angle-side (AAS): two angles and a non-included side of each triangle are equal.

Angle-side-angle (ASA): two angles of each triangle and their included side are equal. State what additional information is required in order to know that the triangles are.

Side-angle-side (SAS): two sides of the triangle and their included angle (the angle between the two sides) are equal in both triangles.

Side-side-side (SSS): both triangles have three sides that equal to each other.

Once you have identified all of the information you can from the given information, you can figure out which theorem will allow you to prove the triangles are congruent. There are five theorems that can be used to prove that triangles are congruent. Choose the correct theorem to prove congruency.